Ratio, Proportion & Partnership Study Material FOR RRB
ssc preparation, important competetion questions, current affairs of india, latest question asked in competetion papers,
 PROPORTION
PROPORTION



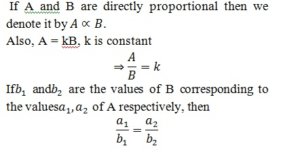 Some Examples:
Some Examples:



Ratio, Proportion & Partnership Study Material
RATIO
Ratio is strictly a mathematical term to compare two similar quantities expressed in the same units. The ratio of two terms ‘x’ and ‘y’ is denoted by x:y.
In general, the ratio of a number x to a number y is defined as the quotient of the numbers x and y.
The numerator of the ratio is called the antecedent (x) and the denominator is called consequent (y) of the ratio.
COMPARISON OF TWO OR MORE RATIOS
Two or more ratios may be compared by reducing the equivalent fractions to a common denominator and then comparing the magnitudes of their numerator. Thus, suppose 2:5, 4:3 and 4:5 are three ratios to be compared then the fractions and are reduced to equivalent fractions with a common denominator. For this, the denominator of each is changed to 15 equal to the L.C.M. their denominators. Hence the given ratios are expressed and or 2:5, 4:3, 4:5 according to magnitude.
Example : Which of the ratio 2:3 and 5:9 is greater?
Solution:
In the form of fractions, the given ratios are 2/3 and 5/9, Reducing them to fractions with a common denominator they are written as 6/9 and 5/9
Hence the greater ratio is 6/9 or 2:3.
Hence the greater ratio is 6/9 or 2:3.
Example: Are the ratio 3 to 4 and 6:8 equal?
Solution:
The ratio are equal if 3/4 = 6/8.
These are equal if their cross products are equal; that is, if 3×8 = 4×6. Since both of these products equal 24, the answer is yes, the ratios are equal.
Remember to be care full order matters! A ratio of 1:7 is not the same as a ratio of 7:1.
REMEMBER
- The two quantities must be of the same kind and in same unit.
- The ratio is a pure number, i.e. without any unit of measurement.
- The ratio would stay unaltered even if both the antecedent and the consequent are multiplied or divided by the same number.
Compound ratio:
Ratios are compounded by multiplying together the antecedents for a new antecedent and the consequent for a new consequent.
The compound of a: b and c: d Is a*c/b*d, i.e., ac: bd.
The compound of a: b and c: d Is a*c/b*d, i.e., ac: bd.
Download RRB JE Study Material
Example : Find the compound ratio of the four ratios: 4:5, 15:13, 26:3 and 6:17
 PROPORTION
PROPORTION
When two ratios are equal, the four quantities composing them are said to be in proportion.
If , then a, b, c, d are in proportion.
This is expressed by saying that ‘a’ is to ‘b’ as ‘c’ is to ‘d’ and the proportion is written as
a:b::c:d or a:b=c:d
The terms a and d are called the extremes while the terms b and c are called means.
TO FIND THE MEAN PROPORTIONAL
Example : Find the mean proportional between 3 and 75.
Solution:
Let x be the required mean proportional. Then, 3:x::x:75 ∴x=√(3×75)=15
Example: A courier charge to a place is proportional to the square root of the weight of the consignment. It costs ` 54 to courier a consignment weighing 25 kilos. How much more will it cost (in rupees) to courier the same consignment as two parcels weighing 16 kilos and 9 kilos respectively?

TO FIND THE VALUES OF AN UNKNOWN WHEN FOUR NUMBERS ARE IN PROPORTION
Example: What must be added to each of the four numbers 10, 18, 22, 38 so that they become in proportion?
Solution:
Let the number to be added to each of the four numbers be x.
By the given condition, we get (10+x):(18+x):(22+x):(38+x)
⇒(10+x)(38+x)=(18+x)(22+x)
⇒380+48x+x^2=396+40x+x^2
Cancelling x^2 from both sides, we get
380+48x=396+40x
⇒48x-40x=396-380
⇒8x-16x=16/8=2
Therefore, 2 should be added to each of the four given numbers.
Let the number to be added to each of the four numbers be x.
By the given condition, we get (10+x):(18+x):(22+x):(38+x)
⇒(10+x)(38+x)=(18+x)(22+x)
⇒380+48x+x^2=396+40x+x^2
Cancelling x^2 from both sides, we get
380+48x=396+40x
⇒48x-40x=396-380
⇒8x-16x=16/8=2
Therefore, 2 should be added to each of the four given numbers.
TO FIND THE FOURTH PROPORTIONAL
Example: Find the fourth proportional to p^2-pq+q^2,p^3+q^3,p-q

USING THEOREM ON EQUAL PROPORTION

DIRECT PROPORTION
If on the increase of one quantity, the other quantity increases to the same extent or on the decrease of one, the other decrease to the same extent, then we say that the given two quantities are directly proportional.
 Some Examples:
Some Examples:- Work done number of men
- Cost number of men
- Work wages
- Working hour of a machine fuel consumed
- Speed distance to be covered
INDIRECT PROPORTION (OR INVERSE PROPORTION)
If on the increase of one quantity, the other quantity decreases to the same extent or vice versa, then we say that the given two quantities are indirectly proportional.

Some Examples:
- More men, less time
- Less men, more hours
- More speed, less time
- More speed, less taken time to be covered distance
RULE OF THREE
In a problem on simple proportion, usually three terms are given and we have to find the fourth term, which we can solve by using Rule of three. In such problems, two of given terms are of same kind and the third term is of same kind as the required fourth term. First of all we have to find whether given problem is a case of direct proportion or indirect proportion. For this, write the given quantities under their respective headings and then mark the arrow in increasing direction. If both arrows are in some direction then the relation between them is direct otherwise it is indirect or inverse proportion. Proportion will be made by either head to tail or tail to head.
The complete procedure can be understood by the examples.
Example: A man completes 5/8 of a job in 10 days. At this rate, how many more days will it takes him to finish the job?

PARTNERSHIP
A partnership is an association of two or more persons who lives invest their money in order to carry on a certain business.
A partner who manages the business is called the working partner and the one who simply invests the money is called the sleeping partner.
Partnership is of two kinds:
(i) Simple
(ii) Compound
Simple partnership: If the capitals is of the partners are invested for the same period, the partnership is called simple.
Compound partnership: If the capitals of the partners are invested for different lengths of time, the partnership is called compound.
If the period of investment is the same for each partner, then the profit or loss is divided in the ratio of their investments.
If A and B are partners in a business, then
(Investment of A)/(Investment of B)=(Profit of A)/(Profit of B) or =(Loss of A)/(Loss of B)
If A, B and C are partners in a business, then
Investment of A:Investment of B:Investment of C =Profit of A:Profit of B: Profit of C, or =Loss of A: Loss of B: Loss of C
(Investment of A)/(Investment of B)=(Profit of A)/(Profit of B) or =(Loss of A)/(Loss of B)
If A, B and C are partners in a business, then
Investment of A:Investment of B:Investment of C =Profit of A:Profit of B: Profit of C, or =Loss of A: Loss of B: Loss of C
Example: Divide `581 among A, B and C such that four times A share is equal to 5 times B’s share which is equal to seven times C’s share.

To Get More Examples and Exercise Download PDF…
Comments
Post a Comment